Example-1-
Write the solution set of the equation x2+x-2=0.
Solution-
x2-x+2x-2=0
x(x-1)+ 2(x-1)=0
(x-1)(x+2)=0
x=1 , x= – 2
S={1, -2}
Example-2-
Write the set {x: x is a positive integer and x2<40}
Solution-
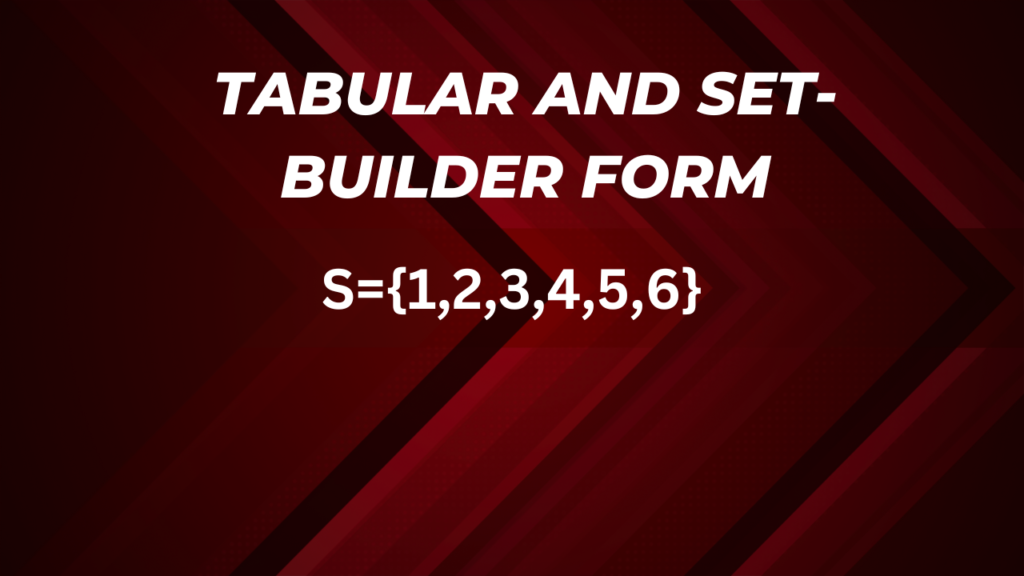
S={1, 2, 3, 4, 5, 6}
7, 8, 9, 10, ………… can not be included because their squares are greater than 40.
Example-3-
Write the set A={1, 4, 9, 16, 25, 36, ………..} in set builder form.
Solution-
S={x: x is square of a natural number}
You can also write the set as
S={x: x=n2, where n ∈ N}
Example-4-
Write the set {1⁄2, 2⁄3, 3⁄4, 4⁄5, ……………………} in the set-builder form.
Solution-
S={x: x=n⁄(n+1), where n∈ N and 1<=n<=6}.
To see the explanation watch the video.