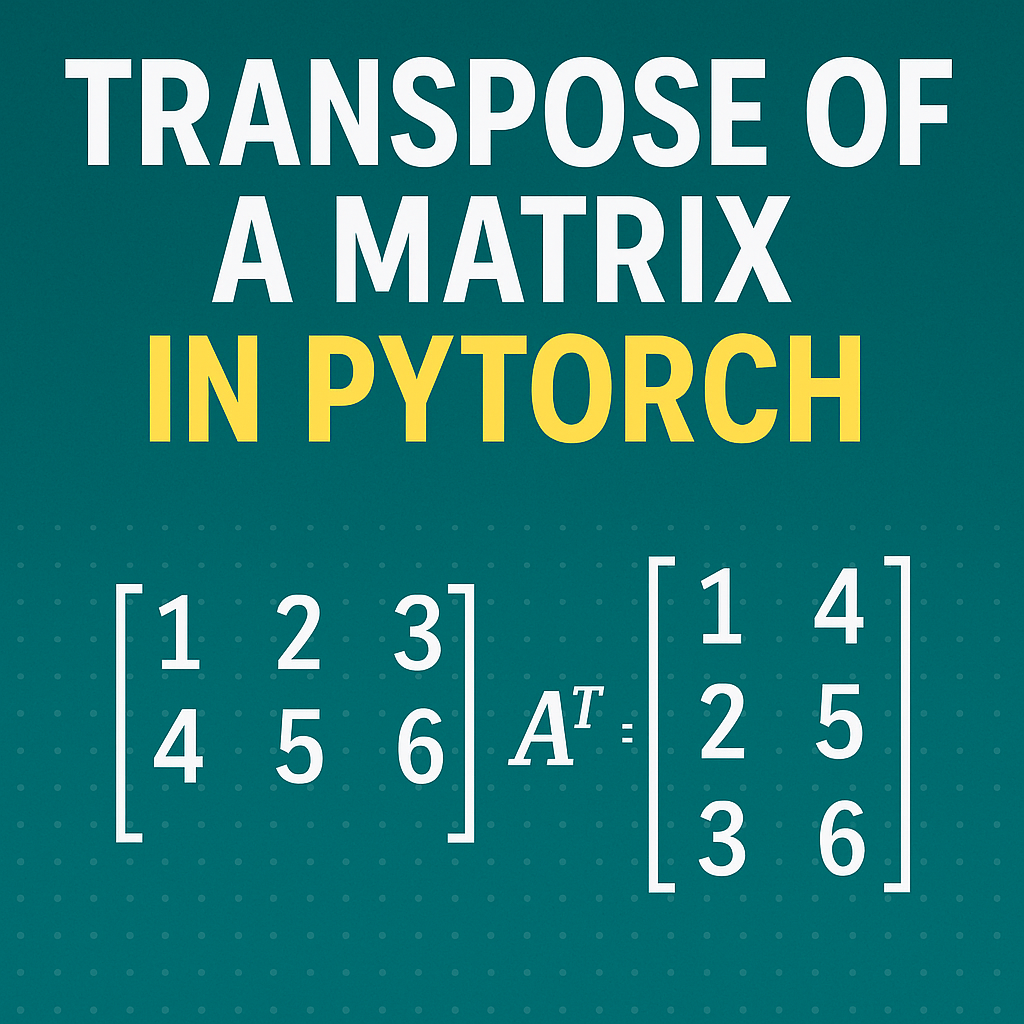
Transpose of a Matrix Author: Bindeshwar Singh Kushwaha Institute: PostNetwork Academy What is the Transpose of a Matrix? The transpose of a matrix \( A \), denoted \( A^T \), is obtained by interchanging rows and columns. If \( A = \begin{bmatrix} 1 & 2 & 3 \\ 4 & 5 & 6 \end{bmatrix} \), […]
Transpose of a Matrix in PyTorch Read More »
Binomial Distribution Data Science and A.I. Lecture Series By Bindeshwar Singh Kushwaha | PostNetwork Academy Binomial Probability Function The binomial probability function is given by: \[ P(X = k) = \binom{n}{k} p^k (1 – p)^{n – k} \] where: \( n \) = total number of trials \( k \) = number of successes
Binomial Distribution Data Science and A.I. Lecture Series Read More »
Bernoulli Distribution Data Science and A.I. Lecture Series By Bindeshwar Singh Kushwaha | PostNetwork Academy Introduction to Bernoulli Distribution A Bernoulli trial is an experiment with only two possible outcomes: Success (1) and Failure (0). If p is the probability of success, then q = 1 – p is the probability of failure. A random
Bernoulli Distribution in Probability and Statistics Read More »
Addition, Multiplication Theorem of Expectation and Covariance Data Science and A.I. Lecture Series By Bindeshwar Singh Kushwaha PostNetwork Academy Outline Introduction Addition Theorem of Expectation Proof of Addition Theorem Multiplication Theorem of Expectation Proof of Multiplication Theorem Covariance Introduction Expectation (or expected value) is a fundamental concept in probability and statistics. It provides a measure
Addition, Multiplication Theorem of Expectation and Covariance Read More »
Moments and Other Measures in Terms of Expectations Data Science and A.I. Lecture Series By Bindeshwar Singh Kushwaha – PostNetwork Academy Moments The \( r^{th} \) order moment about any point \( A \) of a variable \( X \) is given by: For discrete variables: \[ \mu_r’ = \sum_{i=1}^{n} p_i (x_i – A)^r
Moments and Other Measures in Terms of Expectations Read More »
Bivariate Discrete Cumulative Distribution Function Data Science and A.I. Lecture Series Author: Bindeshwar Singh Kushwaha Institute: PostNetwork Academy Joint and Marginal Distribution Functions for Discrete Random Variables Two-Dimensional Joint Distribution Function The distribution function of the two-dimensional random variable \((X, Y)\) for all real \(x\) and \(y\) is defined as: \[ F(x,y) = P(X \leq
Bivariate Discrete Cumulative Distribution Function Read More »
Bivariate Discrete Random Variables Data Science and A.I. Lecture Series By Bindeshwar Singh Kushwaha, PostNetwork Academy Definition Let \( X \) and \( Y \) be two discrete random variables defined on the sample space \( S \) of a random experiment. Then, the function \( (X, Y) \) defined on the same sample space
Bivariate Discrete Random Variables Data Science and A.I. Lecture Series Read More »
Tokens in Python Introduction Tokens are the smallest individual units in a Python program. Everything in a Python program is built using tokens. Python has five types of tokens: Keywords: Reserved words in Python. Identifiers: Names given to variables, functions, and classes. Literals: Fixed values such as numbers, strings, and boolean values. Operators: Symbols
Central Limit Theorem (CLT) and Uniformly Minimum Variance Unbiased Estimator (UMVUE) By: Bindeshwar Singh Kushwaha Institute: PostNetwork Academy Question 1 Suppose \( X_1, X_2, \dots \) is an i.i.d. sequence of random variables with common variance \( \sigma^2 > 0 \). Define: \[ Y_n = \frac{1}{n} \sum_{i=1}^{n} X_{2i-1}, \quad Z_n = \frac{1}{n} \sum_{i=1}^{n} X_{2i} \]
Central Limit Theorem (CLT) and Uniformly Minimum Variance Unbiased Estimator (UMVUE) Read More »
Continuous Random Variable and Probability Density Function Data Science and A.I. Lecture Series Continuous Random Variable and Probability Density Function A random variable is continuous if it can take any real value within a given range. Instead of probability mass function, we use probability density function (PDF), denoted by \( f(x) \). The probability
Continuous Random Variable and Probability Density Function Read More »