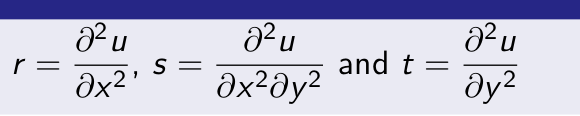Classification of Second Order Partial Differential Equations

If z is a function of two independent variables x and y. Then second order partial differential equation

Rr+Ss+Tt+ f(x,y,z,p,q)=0

Where
r, s and t are

and R, S and T are continuous functions of x and y in domain of xy-plane.

---

Then if
S²-4RT > 0
Then equation is hyperbolic

---

S²-4RT= 0
Then equation is parabolic

---

S²-4RT= 0
Then equation is elliptic

---

Example-

3 u_xx + 6 u_xy + 2 u_yy = 0

R=3, S= 6 and T=2
And S²-4RT= 36 – 24= 12 >0
Then the equation is hyperbolic.